I read something the other day, in material I had paid for which caused my great anguish. In explaining the concept of infinity it was written “[infinity] is the largest number you can possible imagine”. How can one have any hope for the progression of logical thought if comments like this go unchecked within our society? The most fundamental flaw in the statement above is, of course, the inference that infinity has a bound – it is referred to as the largest number, which even a five year old could tell you to add one to. As is often the case when I come across a blatant abuse of mathematics it got me thinking about the subtlety behind it.
One of the earliest discussions of infinity know is the whimsical account of the tortoise and Achilles, set out by the (very) late Greek philosopher Zeno, who supposed that the Greek hero Achilles had a race with a tortoise, and in this race the tortoise was given a head-start on Achilles; such is correct to do, since Achilles is likely to win anyhow right? Now both start moving in the race and and eventually Achilles gets to the point where the tortoise started, but by this point the tortoise has progressed a little bit further. Reset. Achilles arrives at the tortoises next position and alas the tortoise has moved on again. What we see is that the sequence has no end, and whilst Achilles gets closer and closer to the tortoise they never meet. The tortoise wins, the crowds go wild. This result is clearly at odds with observational evidence, the thinkers among you will know why, but it does bring forth the interesting notion of a boundless infinity – something that gets ever larger or in this case ever smaller, but knows no bound. Now if the Greeks could play around with this some 500 years before the birth of Christ, why in the good name of Planet Earth do we need to water down the concept in a text book in 2016?
The area where of course infinity is something where everyone with any basic mathematics had dealt with is that of real analysis; integration being a good example. The very notion of integration is to divide the area beneath a graph up into lots of much smaller areas and then sum these areas to find my total area. What we of course find is that the larger the number of areas we use to divide, the more accurate the result – or to put it another way the smaller the individual areas we are summing together, the more precision in the result. So what we end up with is the below – here we are saying if we integrate a function of t with respect to t between minus infinity and infinity, we get an answer a where a ε R (real number). Does this have to be so? Well no in short – my answer can of course also be infinite, which would imply that there is no bound to the area under the curve – which is okay, you can simply think of that like a very simple quadratic parabola.
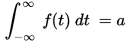
In fact when you really dig down into infinity it finds its way into many areas of mathematics – complex analysis is an obvious choice but there are others such as geometry and topology (the notion of an infinite space). But I do not wish the rest of this post to be be listing areas where infinity crops up in mathematics, that is fairly dry what I think is of much more interest is to talk about some of the surprising features that infinity throws at us. Indeed infinity can be such a mentally demanding notion that there were many who refused to believe of its existence at all.
If there is indeed this boundless number that never ends, two infinities must indeed have the same size must they not? For indeed if one is larger than the other then one is the true infinity and the other is not (there is a fairly fundamental issue with regarding infinity as “having size” but roll with it here). Now let us take some sets of numbers – we are mathematicians and we like sets of numbers. Let us take the first set of numbers and dub them the counting numbers. This set is nothing to be scared of, it is simply the set {1,2,3,4… ∞}. We have left out zero and negative numbers – taking only the real positive integers. This is fine – when it’s your set you do what you like. Now let us take another set, this set is going to be {2,4,6… ∞}, or in other words all positive even numbers and what we can quickly deduce is that the second set is a subset of the first. But hold on now – we have an issue here; if the second set is contained within the first set when I write all of the numbers out I can map every single member of my second set to my first set. If both sets have no bound, can you see the issue? They should indeed be the same size since they are both infinite however set 2 fits inside set 1 and leaves out half the numbers – set 1 should indeed be a larger infinity. Is that okay?
Well this was the logic used by Cantor, who revolutionized the way in which we think about infinity and more specifically number sets. In turns out we shouldn’t be so scared of having large and small infinities. And there are situations where we get even bigger infinities still – say for example in the above… what if we kept our counting numbers but then we considered all of the known fractions? There are some horrendous fractions out there.. the square root of 2 to name a particularly problematic one. What we soon find is that we cannot have a 1:1 mapping like we could before and we have a very large infinity indeed. One of Cantors most interesting and perhaps most famous results was to show that the real numbers are the most numerous natural numbers. That might not seem like much but I can assure you it is quite something to be able to rank sets with no bound by size.
Now let us come back to where we started – with that treacherous statement. I hope you can see why we must never refer to infinity as the largest number we can imagine – indeed there is a fundamental problem with imagining infinity at all for humans, we can only ever comprehend it by reference to something finite – e.g. by taking a number and adding one more endlessly, but by doing this we have infringed on some of the subtlety that infinity has to offer us. If you now think you understand infinity you probably missed the point somewhere along the way. The very notion fills a physicist with dread. We can show lots of things when considering infinity – for example I can demonstrate to you that should space be infinity then with no expansion space would still collapse in on itself with it looking as though it were collapsing into a center point for each observer – that’s interesting. But there is a fundamental barrier to human understanding – if space is not infinite then what is outside it’s bound? Nothing? What is nothing? If it is infinite, why do theories like the inflationary universe theory seem so accurate? I leave you to ponder.

Many things spring to mind- namely “would imply that there is no bound to the area under the curve”- wrong- the fact that the area is under a curve immediately states a finite area. If it wasn’t so there could be no definite answer. All mathematics begin with the natural world and its order. And I completely agree that that starting statement is the worst definition of Infinity ever.
The true problem with infinity is this: we only say ” if space is not infinite then what is outside it’s bound?” because for us, everything that exists has a finite dimension. But by analysing true natural numbers you realise that no half apples ever grew on a tree. Things do not move from 0 to 1 by increments they already are 1. Putting a 0 into mathematics provides us with the boundary that we need to measure by and hence think that in the far of distance there is infinity.
Great piece by the way found it very thought provoking – also wrote an article on the Zeno paradox if you have time to read it.
LikeLiked by 1 person
Thank you very much for your comments and your kind words!
The only thing I will have to disagree with you on is your conjecture that the fact that the area is under the curve immediately states it is finite. This is would violate the Cantorian logic, because we would say because the even numbers are within the integers we immediately take them as finite. The logic is actually simpler than that – when we sum across the entire axis we have two possibilities – the area has a bound or the area has no bound. That is a sum from minus infinity to infinity which either produces a finite area or an infinite one – i.e. one with no bound.
I will certainly check out your post now, thank you for stopping by!
LikeLiked by 1 person
I vaguely remember integration at school. Lol I am finding the statement “the area has no bound” makes no sense; because you use the word area then a finite number or space is implied. Once you try to think of numbers or space going on forever, then in some essence you have missed the idea of infinity.
LikeLiked by 1 person
Agreed – but rather think of the area as the notion of space, where in this instance we need one boundary (the curve) but the space isn’t closed – so we can easily define if something is “in” the area or “out” of the area but the area itself has no bound. Infinity and the real world is mind blowing.
LikeLiked by 2 people
Can I just say, that there is nothing out there able to measure the surface area of belly button fluff. There, I’ve said it and now it is captured in time and space on a comment to rationalise the universe, woohoo! 🙂
LikeLiked by 3 people
I mean… where do I go from here? I don’t know what to say except give you much praise for completely ambushing my mind. Prepare to spend the next hour thinking about belly buttons.
LikeLiked by 3 people
My work here is done 😀 {{{giggles}}}
LikeLiked by 1 person
Ponder I shall, being as I would not categorise myself as a Physics or Maths guru; however both do intrigue me. I for one would like to know what lies beyond a space that cannot be considered infinite; quite the mystery (perhaps)? I finally add that I have enjoyed reading, what I truly consider to be a riveting post. How great it is to be able to actually read such thought provoking material here on humble WordPress. Thanks!
LikeLiked by 2 people
Most of the people who have made the most significant break throughs would not of considered themselves gurus either so I wouldn’t worry about that. Thank you so much for your kind words, I am glad you found it interesting but the credit must go to nature – I defy a human not to be captivated by the infinite
LikeLiked by 3 people
Agreed upon that – often knowing too much clouds your ability to perceive change or provide creative responses outside of the box. I may not be a guru, but I certainly know plenty, and have learned plenty! Yes, it is no doubt the unknown quality that attracts interest, or the unknown possibility! Thanks again, Bex
LikeLiked by 1 person
Pingback: News and musings – Adventure and Business·
Thanks for the interesting article. Infinity involves a lot of subtle difficulties. Arguably, mathematicians haven’t finished sorting out all those subtleties (confer the Banach-Tarski Paradox).
LikeLiked by 2 people
Really enjoyed reading this post …what I’m finding a bit weird tho is that I personally find the notion of infinity reassuring …is that weird? ….I just feel it’s as is ….but then again my brain is akin to Winnie the Pooh:D:D:D
LikeLiked by 2 people
I mean the idea of anything not ending i think is in a way comforting to the human experience right – because the thing we all don’t like is the fact that it must come to an end
LikeLiked by 2 people
Hmmmm …one to ponder …I guess I just like to try and enjoy this ride and we’ll see what’s coming next ….it could be a great adventure
I quite fancy coming back as a cosmic ray …or at the very least a domestic cat …..wouldn’t fancy being a slug tho or WORSE a bluebottle …have you seen the film ‘The Fly’? …..it freaked me out …that little head saying ‘Help Me ….bzzzzzzzzz’ it’s making me shudder now:D:D:D
LikeLiked by 1 person
I have just studied infinity and integers. I have a question though, is the continuum hypothesis considered a part of the infinity or more than infinity? As I have read about the CH I have found, like infinity, there are no “ends”. Objects were used instead of numbers to show continuum hypothesis.
I don’t have a good understanding of math but I try!
LikeLiked by 1 person
What a beautiful question for a Tuesday afternoon. As far as I understand, the whole idea is comparing the cardinality (size) of the two sets… so {1,2,…26} has the same cardinality as {a,b,…,z} because I can map 1-a, 2-b,…,26-z.. it is a 1:1 relationship. Now the continuum hypothesis is the heart of Cantor – how can we speak of cardinality of an infinite set it gets more interesting. The continuum hypothesis shows that in practice any set of reals has a size equal to either the reals or the integers. It is really interesting and it raises a rather subtle question: can one have a large and a small infinity? Many people would say no, by definition you can’t. I say that if in practice you can, your definition is not correct. So basically – it is part of infinity!
LikeLiked by 1 person
What I understood about infinity to the smallest is using -1, -2,… The CH is fascinating because it brings in the philosophy of math! It’s called the provable unsolvable!
LikeLiked by 2 people
Indeed the philosophy of mathematics is truly quite mind-bending, because it takes things that you laid down as fact early on in your academic life and then drags them out into question
LikeLiked by 2 people
There are actually different levels of infinity. Countable infinity (aleph 0) is the lowest level, and can be thought of as discreet infinity. Continuous infinity (aleph 1) can be proven to be unmappable to countable. Any countably infinite set can be mapped one-to-one from the natural numbers. For example natural numbers map to whole numbers like so: 1 -> 0, Nn -> Nn-1. Integers like this: 1 -> 0, Nn -> floor(Nn / 2) * (1 – 2 * (Nn modulo 2)). No such mapping exists for the real numbers, which are continuously infinite. The proof is known as Cantor’s diagonal argument.
LikeLiked by 1 person
Very interesting – it is an area which I think people are guilty of looking at with too much simplicity…. it really is very complex. I have read various bits and pieces about and by Cantor he really does have a very interesting mind
LikeLike
Achilles and the Tortoise brings back fond memories of “Godel Escher Bach”.
LikeLiked by 1 person
An interesting example of non-intuitive infinity is the coastline paradox: “The length of a ‘true fractal’ always diverges to infinity; if one were to measure a coastline with infinite, or near-infinite resolution, the length of the infinitely smaller bends of the coastline would add up to infinity.”
LikeLiked by 1 person
I love infinity paradoxes!
LikeLike
In simple terms, infinity can be defined as as that which is bigger than the biggest. Now let us analyse what it means. I imagine a number which very very large. Now according to my definition, infinity will be bigger than that imagined number. That means I have thought a number which is bigger than the biggest. Now that number which is thought by me becomes the biggest number. Again applying my definition, infinity will be bigger that that thought number. Again the same procedure applies. This procedure will continue, endlessly. Or it is a sort of never ending loop. This is the way to define infinity. Pure infinity is not reachable but it continues increasing,
Mathematically, I imagine a number p which is infinite. Then according to my def , another number which is greater than p will be infinity. Let this number be q.
Then q > p, Again q becomes greatest number. Then according to my def
another number r is greater than q, or
r > q
Similarly, s > r
This will proceed endlessly to find the number infinity.
This is my perception of infinity.
I have not used jugglery of words and it is very simple.
Do you approve it?
Although infinity is endlessly large but it is obtained from real numbers as you explained summation of 1+2+3+4+. up to infinity is infinity.
Or tan pi/2 or 1+1/2+1/3+1/5+———
If we expand log(1+x), it is x-square x /2 + cube x/3 so on
Now if we put x= -1 then
It becomes log(1-1) = 1+1/2+1|3 so on
= Log 0 = – Infinity
That means infinity is function of real number.
Also i raised to power i has its principal value which is real.
where i is square root of -1 .
I have also dealt complex number in an article in very simple terms. If you find time, you may go through it at https://narinderkw.wordpress.com/2016/06/22/revolutionary-approach-to-solving-indepently-problems-of-complex-numbers-by-rotating-operator-i/
LikeLiked by 1 person
I do indeed approve of your definition as an iterative process and I think that this will most certainly help you to explore the “biggest” number from that perspective. I do think however the definition needs a little expansion when you consider limits – for example the paradox of Achilles and the tortoise is so because the common misconception is that summing anything to infinity gives an infinitely large number. Sometimes it does not it gives a finite number; this can be difficult for many to get their head around – but really it is just the difference of convergence and divergence. Cardinality in set theory is another worthwhile mention – the idea that two sets can be infinitely large but one larger than the other as proven by Cantor
LikeLike
You rightly said every convergent series does not sum up to fixed value. But is a method to know convergence of a series.
Lt n tends to infinity,
(n+1) th term divided by nth term should be less than one,
Also n tending to infinity, nth term should tend to zero.
These are some the tests for convergence of a series. But these are not always true.
Then comes the method of integration as you beautifully explained in this or other article. If the series is confined in that integration, it is convergent. Here the integration is not accurate because n varies in digits
In such cases, it is better to remember, expansion of series of certain functions like sin, cos, tan, cot, e to power x, a to power x, log(1+x) and inverse functions.
If the series matches with any function, then putting the value in that function and finding the value of function, one can find whether the series sums up to fixed value or not.
Once I was working on how to expand any function in power series without taking the help of Taylor Expansion or Mclaurin series. I did a bit also. Thereafter finding that series, how one can solve differential equations, integration etc.
I was also attempting to find that property of the series which tells it is sinusoidal in nature. And then finding out the criterion from the series to prove that it represents a wave.
LikeLiked by 1 person
Hi Mr Joseph,
You are going great with your articles and it pleases me.
I have also attempted solving 0/0, if you find time from your busy schedule of study and articulation, you may read it. I am writing to you because I liked your article on infinity and zero and infinity are reciprocal of each other, it may seem a but interesting to you. Have a nice time!
Regards.
LikeLike
I have to say on this one i am not sure what you are getting at – I think the basis to understanding why 0/0 is a mathematical indeterminate is understanding where it comes from in the first place – which is the basic definition of multiplication. There isn’t actually an issue with 0/0 – it isn’t a problem that needs solving. I am afraid on this one I cannot agree with your reasoning at all! Still I commend you for thinking through these issues
LikeLike
Since you explained infinity in one article, I referred the issue of 0/0 to you as I had attempted solving it in one of my article. In Physics, mass of photon in motion, takes the form 0/0 but this mass of photon is h.f/c squared in quantum mechanics and is not indeterminate, prompted me to analyse 0/0.
Thanks for your comments. Regards.
LikeLike
I know – I am aware of this, but the mass of a photon isn’t the way it is because of some special phenomena, it is the fact that it does not interact with the Higgs field. They are different things and one does not imply the answer to 0/0. Thank you
Joe
LikeLike
I know of Higgs Boson field which imparts mass to particles due to its interaction. But here the issue was why there arose necessity of solving 0/0. As far as Higgs Bosons field is concerned, I have composed an article on Boson.
Thanks and regards.
LikeLike